Chapter 5

Projections to lines

Let X: Mⁿ -> Rⁿ⁺¹ be an immersion of the smooth n-manifold M into Euclidean (n + 1)-space. For each unit vector V in Rⁿ⁺¹ let _V: M -> R be the height function in the direction of V, the composition of X with orthogonal projection to the line spanned by V:

_V(P) = X(P) ^. V

: Sⁿ x Mⁿ -> Sⁿ x R,   (V, P) = (V, _V(p))

The catastrophe map of the family of height functions on an immersed hypersurface is the Gauss map of the hypersurface. Let : C -> Sⁿ be the catstrophe map of the family . Since (V, P) C if and only if V is normal to X at P, and (V, P) = V, is precisely the Gauss map of X. If M is orientable, the restriction of to each component of C is the Gauss map of X associated with the orientation of M corresponding to that component. If M is not assumed to be orientable, the Gasus map N of X is defined as the map from M to projective space RPⁿ which assigns to each P M the line through the origin in Rⁿ⁺¹ normal to the tangent hyperplane of X at P. We therefore have that s ° = N ° r, where r: Cⁿ -> Mⁿ and s: Sⁿ -> RPⁿ are the canonical double coverings.

We discovered that the Gauss map of a hypersurface is a catastrophe map while we were looking at the theorems on the singularities of the Gauss map proved recently by Menn (unpublished), Bleeker and Wlison [BlW], and Wall [Wa2]. We then learned that this observation had been made by Weinstein in 1969 [We], and more recently by Bruce [Bru1] and C. Romero Fuster [Rom1].

A corollary of this observation is Menn's result that the Gauss map N of a generic hypersurface in R⁴ can have umbilic singularities, so N is not necessarily stable. A more general observation is that Gauss maps of hypersurfaces are Lagrangian maps (cf. chapter 6 below), so they have Lagrangian singularities, and hence they are not stable in general [Wa2]. Arnold has informed us that he observed that the Gauss map is Lagrangian as early as 1966 (cf. [AGV]).

Now we specialize to immersion of surfaces in 3-space. Several of our characterizations of Gaussian cusps are corollaries of the following result.

Theorem 5.1 Let M² be a smooth surface. For an open dense subset A of the space of immersions X: M² -> R³, the germ at (V, P) of the family : S² x M² -> S² x R is a versal unfolding of the germ at P of _V for all (V, P) S² x M².

Proof This is equivalent to the statement that the germ at (V, P) of the mapping is stable, since is a family of real-valued functions. It is easy to check, using local coordinates and Mather's infinitesimal stability criterion [GolG, p. 73], that the germ of at (V, P) is stable if and only if the germ of the Gauss map of X at P is stable. Bleeker and Wilson [BlW] showed that the set of immersions of an oriented surface M² in R³, such that the Gauss map is stable, is open and dense in the space of all immersions of M² in R³. Their proof adapts to show that the set A of immersions of an arbitrary surface, such that the germ at each point of the Gauss map is stable, is also open and dense.

The following corollary implies theorem 3.1(b).

Corollary 5.2 If P is a cusp of the Gauss map of X: M² -> R³, then for each  > 0 there exist three distinct points Q₁, Q₂, Q₃ in M such that |P - Q_i| <  for i = 1, 2, 3, and the tangent plances to X at Q₁, Q₂, Q₃ are parallel. If X A, then cusps of the Gauss map of X are the only points of M with this property.

Proof Let V be a unit normal to X at P. The points Q₁, Q₂, Q₃ are critical points of a height function _W for W near V. But P is a cusp of the Gauss map of X if and only if (V, P) is a cusp of the catastrophe map of the family . For each W S², ^-1(W) is by definition the set of critical points of the height function _W. If (V, P) is a cusp of then there exist W arbitrarily close to V such that ^-1(W) has three points [C2, Fig. 6, p. 773]. On the other hand, if X A then the germ of at a point (V, P) is either regular, a fold, or a cusp. If this germ is regular, then ^-1(W) has one point for W near V. If the germ is a fold, then ^-1(W) has either no points or two points for W near V [C2, Fig. 6, p. 774].

Corollary 5.3 If P is a cusp of the Gauss map of X: M² -> R³, the for each  > 0 there exist two distinct points Q₁, Q₂ M such that |P - Q_i| <  for i = 1, 2, and the tangent planes to X at Q₁ and Q₂ are equal. If X A, then cusps of the Gauss map of X are the only points of M with this property.

Proof Let V be a unit normal to X at P. The points Q₁ and Q₂ are critical points of a height function _W for each W near V, with _W(Q₁) = _W(Q₂). Since is the catastrophe map of the family :S² x M² -> S² x R, the germ of at (V, P) is a cusp (resp. a fold, regular) if and only if the germ of at (V, P) is a swallowtail (resp. a cusp, a fold). The image of the critical set of near a swallwtail has a curve of double points [C2, Fig. 23, p. 784], i.e. there are two curves 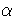, : [0, ) -> C such that 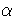(0) = (V, P) = (0), ((0, 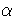))  ((0, )) = Ø and ((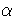t)) = ((t)) for all t in [0, ). The image of the critical set near a fold or a cusp has no double points.

For an arbitrary immersion X: M² -> R³, this bitangent property does not necessarily hold for all parabolic points which are not folds of the Gauss map. For example, consider the surface

X(x, y) = (x, y, x⁴/4 - y²/2)

The bitangent property does hold, however if P is an isolated parabolic point which is a ramification point of the Gauss map (cf. the monkey saddle X(x, y) = (x, y, x³/3 - xy²), chapter 2, example 3).

The results of this section can be interpreted using the classical geometry of contact with planes. The point P is a critical point of the height function _V if and only if the plane through X(P) perpendicular to V is tangent to the immersion X at P. The point P is a degenerate critical point of _V if and only it this tangent plane T_P has unusual contact with X at P. More precisely, there is the following classification of points P M² into three types (I, II, III) for X A.

Let V be a unit normal to X at P, and let (V, P) = {Q M | _V(Q) = _V(P)}, the level curve of _V through P. Notice that (V, P) = {Q M| X(Q) T_P}, the intersection of the surface with its tangent plane at P.

I.

1. P is nondegenerate critical point of _V, so _V has Milnor number one at P.
2. P is a regular point of the Gauss map of X.
3. T_P has ordinary first order contact with X at P.
4. Either has an isolated point at P (X is elliptic at P) or has a node at P (X is hyperbolic at P). Cf. [Sa, 264].

II.

1. P is degenerate critical point of _V with Milnor number two at P.
2. P is a fold of the Gauss map of X.
3. T_P has stationary contact with X at P (cf. [Sa, §§ 204, 266, 269]).
4. has a cusp at P (cf. [HC, Fig. 207, p. 199]). The immersion X is parabolic at P. Cf. [Sa, 264].

III.

1. P is a degenerate critical point of _V with Milnor number three at P.
2. P is a cusp of the Gauss map of X.
3. T_P is a tacnodal tangent plane of X [Sa, § 604].
4. has either an isolated point (an imaginary tacnode) at P (P is an elliptic cusp of the Gauss map), or has a tacnode at P (P is a hyperbolic cusp of the Gauss map).