Figure 5 for Kappraff's "The 'Arithmetic' of Nicomachus of Gerasa and its Applications to Systems of Proportion" in NNJ v2n4

Figures for Jay Kappraff's
The Arithmetic of Nicomachus of Gerasa
and its Applications to Systems of Proportion

| Fig. 1 | Fig. 2 | Fig. 3 | Fig. 4 | Fig. 5 | Fig. 6 | Fig. 7 | Fig. 8 | Fig. 9 | Fig. 10 |

Fig. 5. The geometrical construction referred to by Tons Brunés, a Danish engineer and student of ancient geometry, as the Sacred Cut. When the point of a compass is placed at one vertex of a square and an arc is swept out, the edge of the square is cut by a factor of 1/Ö2, the sacred cut. Four sacred cuts mark the vertices of a regular octagon and lead to a subdivision of the square into three species of rectangles: a square of proportion 1:1; a square root rectangle of proportion 1:Ö2; and a rectangle of proportion 1: q, referred to as a Roman rectangle. | top of page | back to text |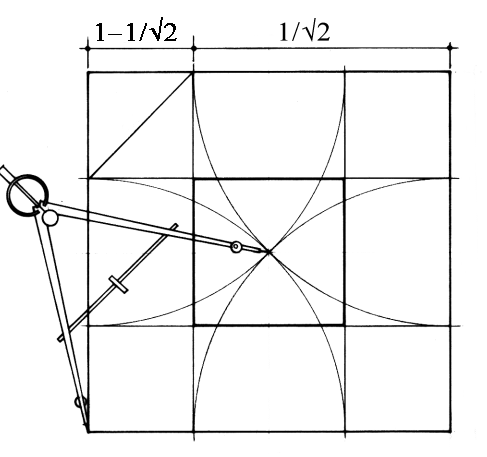

The Sacred Cut construction of Tons Brunés

| Top of Page |