"Dynamical Symmetries: Mathematical Synthesis between Chaos Theory (Complexity), Fractal Geometry, and the Golden Mean" Nigel Reading [Reading 1994]

Reviewed by Michael J. Ostwald and Stephen R. Wassell

While it was written in the mid-1990s, Nigel Reading's paper "Dynamical Symmetries" [Reading 1994] has had a curious and lasting influence on recent architectural theory. This is perplexing because his paper is one of the very few published in a mainstream journal of architecture (Architectural Design) that actually features basic mathematical formulas and notations. The paper is also densely written in a mixture of scientific and mathematical terminology (or, perhaps more accurately, metaphysical and pseudo-mathematical jargon) making it difficult for the average architectural reader to decipher.[1] Despite this relative obscurity, the journal is widely read by young architects and thus, Reading's paper has become regularly cited by designers and students studying the connection between architecture, aesthetics and nature.

Reading seeks to inform architectural design through the study of dynamical systems theory, in particular by considering the way in which complex patterns form in the zone between "ordered, stable systems at equilibrium (high entropy) and disordered and unstable Chaotic (low entropy) ones" [Reading 1994: xii] - a region described variously as the zone of emergence or as the edge of chaos.

This transition zone is occupied mathematically, by the Golden Mean. This ratio acts as an optimised probability operator, like a binary switch, whenever we observe the progressive evolution of a dynamical system. … As far as architectural application is concerned, we must look at the temporal as well as the spatial, at how quite literally, the dynamics (of systems applications) can inform the statics (forms) of building. The aesthetics of the banal imitation of some motif of fractal geometry is simply missing half the picture! [Reading 1994: xii].

It will be useful for us to focus on what Reading states in the last sentence of the above quote; since we will refer to this sentence repeatedly below, let us give it a name: the "banality lament".

Benoit Mandelbrot [1986] concluded, years before architects and artists became interested in the topic of fractal geometry, that a connection does exist between the Golden Mean and fractal geometry but that this connection is limited and largely meaningless. Still, others would undoubtedly disagree; see, for example [de Spinadel 1998]. In any case, although Reading most certainly associates the Golden Mean with fractal geometry in the body of his essay, in the banality lament he seems to be indicating his rejection of the notion that architecture can be meaningfully informed by merely incorporating fractal geometry in some "banal" way. This idea deserves further discussion.

One example of banality would be the dubious assumption that if the Golden Mean is related to fractal geometry, then architecture modelled on the Golden Mean must be natural, because fractals are natural, where "natural" means connected to forms from biology, meteorology, geography, etc. There are two serious problems with this assumption. In the first instance, it assumes that fractals are the geometry of nature whereas, in reality, fractals simply simulate the geometry of nature. This subtle but critical point is often forgotten in the fractal geometry debate, and it completely undermines any supposed natural authority gained by the use of fractal forms in architecture. In essence this is the Kantian aesthetic argument, that there is a "timeless-truth-to-nature", applied to fractal geometry. Yet, as Noel Gray pointed out in 1991, this argument cannot be extrapolated to mathematical models, and certainly not, as Mandelbrot does, to fractal geometry [Gray 1991]. In the second instance, it assumes that the use of the Golden Mean in architecture implies that a building is natural in some way. Yet, any relationship between architecture and nature, by way of the Golden Mean, is abstract not literal. Some architects maintain that the Golden Mean embodies an aspect of natural beauty, as exemplified Platonically in mathematics. They do not argue, however, that architecture based on the Golden Mean is genuinely closely aligned with nature. If a connection exists at all it is symbolic or metaphoric. Thus the proposition that architecture created through manipulations of the Golden Mean takes account of the complexity of nature because of its relation to fractal geometry is flawed on two accounts.

Given Reading's banality lament, one would assume that he intends to avoid such flawed notions. Presumably, then, Reading means to discuss more meaningful ways in which the Golden Mean, as related to chaos theory and fractal geometry, can inform architectural design. He attempts to do this at the end of his essay, as we will discuss below.

First, however, Reading spends the majority of the essay describing the important role of the Golden Mean in dynamical systems. His basic thesis is that the Golden Mean occupies a prominent place at the "edge of chaos" (a term that he highlights in boldface three times in his paper) and thus regulates - even reigns supreme over - the transition between order and chaos, while also somehow marrying the linear and non-linear realms of dynamical systems. Unfortunately, his mathematical descriptions are vague at best, often devoid of substance, and sometimes simply incorrect.

In the first paragraph that includes mathematical symbols, Reading introduces the Golden Number (referring to it by the commonly used symbol f and name Phi) and provides some examples of its "unique qualities". For example, he states, "if one divides Phi by its reciprocal you derive its square:
f /(1/f) = f²" [Reading 1994: xii].

While this equation is certainly true, it is by no means unique to the Golden Number; indeed any number divided by its reciprocal is its square! [2]

The next paragraph that involves numbers is, in its entirety:

The feedback loop which describes Phi is an arithmetic linear operator, (like a binary switch: 0 or 1, off or on) representing the winding or rotation number of the inscribed spiral, which is conventionally represented as a multiple of 2p. This is superposed with its expansion ratio; 1 : 1.618 … which is quadratic (logarithmic) and therefore non-linear. The former is reversible (and finite) while the latter is not (and infinite - being numerically irrational) [Reading 1994: xiii] (original emphasis).

There are various individual words and phrases here that make sense; Reading has clearly familiarized himself with some of the "buzzwords" of dynamical systems theory. However, the non-mathematically-oriented reader who does not understand this paragraph may be surprised to learn that a mathematician would not be much better off! [3] Later in the essay Reading finally gives some details. Unfortunately, several of those details are incorrect; however, so as not to get bogged down in the mathematical pitfalls of Reading's article (because there is another major pitfall to uncover), further examples of inaccuracies are left to the Appendix, as is a basic explanation of the underlying example of chaos.

While metaphors and pseudo-mathematics are prominent throughout Reading's essay, let us assume for the sake of argument that his forays into dynamical systems are actually impeccably correct. Where does that leave us at the end of his essay? Unfortunately, it leaves us with a barrage of vague and imprecise "applications" of the Golden Mean to architecture.

The last page of Reading's four-page essay begins with the paragraph:

It seems clear now that the Golden Mean can certainly be reconciled with the new science, which reveals a profound dynamical aspect to its action. Without prolonging this review excessively, here are some brief examples of how the assimilation of dynamical systems knowledge, married with new engineering, materials and systems technology, might reinvent the statics of architecture [Reading 1994: xv] (original emphasis).

Reading's key lessons for architecture include the ideas that "[c]limate control will increasingly become a hybrid between active elements, such as mechanical ventilation, and the passive, such as natural ventilation"; that facades incorporating electrochromic glass may be linked to a computer that can adjust "facade opaqueness"; that facades will be constructed "using liquid crystal technologies" which can "convey graphic information on the skins of buildings for the external world to observe"; and that "[c]hameleon buildings will for example, be able to react to passing clouds, altering facade U-values and values for solar gain" [Reading 1994: xv]. What exactly all of this has to do with the Golden Mean is unexplained and completely unclear. To progress from a study of a mathematical entity (that has a historic, although metaphoric, connection to architecture) in order to suggest ways in which architecture can learn from dynamical systems seems quite farcical.[4] There is nothing explicitly wrong with his suggestions (most have existed for a number of years); it is the (unexplained) way in which they somehow arise from a study of the Golden Mean that is problematic. This brings us back full circle to Reading's banality lament from the beginning of his article. Even if Reading's mathematical analyses were all correct, his proposals for the application of the Golden Mean to architecture are just as metaphorical and shallow as the "banal imitation of some motif of fractal geometry" that Reading seems to disdain!

Granted, the use of the Golden Mean to regulate the proportions of a building has potential merits. Moreover, the exploration of "green" architecture is certainly meritorious, and dynamical systems theory, as well as other methods of mathematical physics, will undoubtedly be used in the development of suitable environmentally-minded building technologies. In fact, the Golden Mean may very well play some sort of role here, at least inherently in the mathematics. Reading's essay, however, does not meaningfully address these issues, and it should not, in our opinion, be on any recommended reading lists, except perhaps as a reminder to architects and writers that a vast chasm separates symbolic and metaphoric discussions from actual design methodology.

APPENDIX
Reading states:

The one-step feedback loop derived from the iterator: X = X²+ c (the equation for a circle - a section of the Phi temporal evolution cone) with , produces what is known as the super-attractive case. Here two fixed values rather than the normal one are produced, being: 0 and -1. In the case of any other c-value the iterations of feeding the product back into the equation rapidly produces what is the hallmark of Chaos; sensitive dependence on initial conditions - or exponential error propogation! [Reading 1994: xiii] (original emphasis).

Errors: 0 and -1 are not fixed values but rather a 2-cycle, and there are, in fact, an infinite number of c-values that do not produce chaos. In order to understand this, let us consider this rich subject in a little more detail. One of the most basic ways to arrive at an example of chaos is to consider the function F(x) =x² + c. (One could use other functions, but this one is quite simple, and it does lead to chaos.) Here, c is a value that can be chosen as desired; for any fixed c, we can create a "feedback loop" as follows. Pick an initial input (usually called x₀) and plug it into the function to obtain the output (x₀)² + c, which we can call x₁. Now comes the feedback part: take the output, x₁, and use it as a new input (i.e., compute F(x₁) = (x₁)² + c), and call the new output x₂; then feedback again, etc. Let us do an example that will also show why Reading's statement about 0 and -1 is incorrect. Take c = -1 (more on this choice below), so that our function is F(x) = x² -1. Now use x₀ = 0 as the initial value. Then we plug in to get F(0) = 0² -1 = -1. Now take the output, -1 , and plug it in: F(-1) = (-1)² -1 = 0; we return to the initial value! Clearly, if we feedback repeatedly, we'll just get the "2-cycle" 0, -1, 0, -1, …. Contrast this situation to a fixed value, which is a value x such that F(x) = x, i.e., the output is just the same as the input, so the feedback loop will simply give the same value over and over again (hence the term "fixed point"). In fact, we can find a fixed point by simply demanding that x = F(x), i.e., x = x² + c (which is where Reading gets his "iterator"). Using our choice for c, this becomes x = x² - 1, which has two solutions, f and -1/f. So that's where the Golden Mean comes into the picture.

Reading continues:

Remarkably, even if we alter values for x₀ we still derive the same results. This makes the cycle as resistant to permutation as is possible; 0 and -1 represent the repelling fixed points for the equation which in turn, generate a super-attractive orbit, between them [Reading 1994: xiii].

Errors: 0, -1 represents an attractive 2-cycle, whereas f and -1/f are each repelling fixed points. This deserves further consideration. We know that if we start with x₀ = 0, we'll get the 2-cycle 0, -1. But if we start with a value close to 0, say 0.1, we'll get the "orbit":

(the last three values are rounded off to the nearest millionth), which is clearly "converging" to the 2-cycle 0, -1. This is why the 2-cycle is called attractive. On the other hand, let's consider the fixed point f. The fact is that if we start close to f (but not on f), the orbit will be repelled away from f. (In fact, it turns out that if we start just less that f, the orbit will converge to the attracting 2-cycle 0, -1; whereas if we start just greater than f, the orbit will "diverge" to +¥.)

Let us consider one more error of Reading, which will also lead to a discussion of how chaos is obtained:

The constant c = -1 will rapidly produce error if even infinitesimally deviated from, and so represents an island of perfect stability surrounded by a seething maelstrom of Chaos [Reading 1994: xiii].

Wrong again: the constant c = -1 sits right smack in the center of the largest "period-2 bulb" of the Mandlebrot set - the black circle just to the left of the main black area (see the second figure on page xiv; incidentally, Reading's choice of figures for this article is as pseudo-scientific as his writing style). To understand what this means and thus why Reading is incorrect, let us finally discuss how chaos is produced from the dynamics of the function F(x) = x² + c. To do this, we consider what happens as we change the value of c! For certain c-values, we may have some combination of fixed points, 2-cycles, 3-cycles, etc., but for other choices of c, we find that starting with an initial value, say x₀= 0, will result in an orbit that just bounces around in a seemingly random manner - chaos! (There are other important aspects to chaos as well, but at least this provides a general picture. For an understandable account of the details, see [Devaney 1992].) Reading's claim that c-values just off of -1 will lead to chaos, however, is incorrect. Any c-value within 0.25 of (i.e., -1.25 < c < -0.75) will, in fact, lead only to an attracting 2-cycle and two repelling fixed points, just as with the value c = -1[Devaney 1992: 56]. So this is not an example of the Golden Mean sitting at the edge of chaos, as Reading seems to think. He may be getting mixed up between the "period-doubling route to chaos" (the present situation) and the "Golden Mean winding number route"; see [de Spinadel 1998: 101ff.].

NOTES
[1] In fact, the material is written in such an imprecise, fuzzy manner that I (throughout the footnotes, the first person singular tense refers to Steve Wassell) found it challenging to decipher much of the pseudo-mathematics, even concerning material in which I am quite well versed! (It does not help that, although there is a bibliography at the end, nowhere in the paper is there a specific reference to any of the sources.) At first, I wondered if Reading is simply far beyond me, mathematically, but I soon realized that this is not the case; see more on this in later notes. return to text

[2] In other words, for any real number x, it is a simple fact that 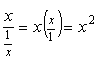. The fact that Reading lists this tautology as a unique property of the Golden Number, while excusable as a simple error, indicates to me that when he later states mathematical concepts that I cannot understand or have never encountered, I should regard them as metaphorical at best - but probably just metaphysical or pseudo-mathematical. return to text

[3] I suppose the only real error here is that "quadratic" and "logarithmic" are two totally different types of behavior, but I would also stress that the various mathematical concepts are not connected together as to make any sense. return to text

[4] Jencks [1995] makes the same mistake. return to text

REFERENCES:
Devaney, Robert L. 1992. A First Course in Chaotic Dynamical Systems: Theory and Experiment. New York: Addison-Wesley.

Gray, Noel. 1991. Critique and a Science for the Sake of Art: Fractals and the Visual Arts. Pp. 317-320 in Leonardo 24: 3.

Jencks, Charles. 1995. The Architecture of the Jumping Universe: A Polemic, How Complexity Science is Changing Architecture and Culture. London: Academy Editions.

Mandelbrot, Benoit B. 1986. Fractals and the Rebirth of Iteration Theory. Pp. 151-160 in The Beauty of Fractals. Heinz Otto Peitgen and Peter H. Richter, eds. New York: Springer-Verlag.

Reading, Nigel. 1994. Dynamical Symmetries: Mathematical Synthesis between Chaos Theory (Complexity), Fractal Geometry and the Golden Mean. Pp. xii-xv in Architectural Design 64: 11/12.

Spinadel, Vera W de. 1998. From the Golden Mean to Chaos. Buenos Aires, Argentina: Nueva Libreria S.R.L.


ABOUT THE REVIEWERS
Michael J. Ostwald is the Book Reviews editor for the Nexus Network Journal. Stephen R. Wassell is the Mathematics editor for the Nexus Network Journal.

Copyright ©2002 Kim Williams