
|
Rudolph M. Schindler: Space Reference Frame, Modular Coordination and the "Row" |
|---|
School of the Arts and Architecture
University of California Los Angeles
Los Angeles, CA 90024 USA
INTRODUCTION
The approach to architectural dimensioning
taken by Rudolph Schindler (1887-1953) is described by Jin-Ho
Park in a complimentary paper in this issue of the Nexus
Network Journal [2003]. While Schindler's "space reference
frame" is becoming better known [Schindler 1946], its relationship
to the "row" is only to be found in Park's recent investigation.
The theory of the "row" counters traditional proportional
notions, many of which are derived from the principle of geometric
similitude: a principle which is mostly represented in architectural
drawings by regulating lines and triangulation. Park gives examples
of this. Here, the simple mathematics of row theory is presented.
A short background note concludes the paper.
SPACE REFERENCE FRAME
Mentally, Schindler [1946] conceived of
a design, within a three-dimensional integer lattice, or Cartesian
grid:
As an architect, Schindler had to choose a suitable module for his "unit." In most of his designs, he chose 48 in. This module might be divided, Schindler argued, by 1/2, 1/3, 1/4, without losing a "feeling" for the dimensions. In fact, Schindler used 36 in., 24 in. and 12 in. as refinements in plan, and the one third divisions, 16 in. and 32 in., almost exclusively in elevation.
Consider a general module M. The equivalent divisions for dimensional refinements are then M/4, M/3, M/2, 2M/3, 3M/4; but clearly this could be further generalized.
INTRODUCING THE ROW CONCEPT
In his unpublished
1917 Emma Church School lecture notes, Schindler cryptically
defined a row as a "following of unequal units with definite
changes [of] relation." He then gave this example:
After Robinson [1899], he suggested an interpretation for this sequence, or row, as intervallic ratios in a musical scale. Schindler suggested the general rule. If p/q is a term in a row, then the successor term is given by the rule:
Thus, (p - 1)/(q - 1), p/q, (p + 1)/(q + 1) are three successive terms in a row, providing p, q > 1.
Schindler then gave another, abbreviated example:
1/3, ... 11/13,
while Robinson, in addition, set out the rows beginning with 1/4 and 1/5.
The fractional notation, p/q, was used by both Robinson and Schindler. Each row may be depicted as a sequence of rectangles by setting p/q as the vertex (p, q), and the other vertices as (p, 0), (0, q), (0, 0). This produces a diagram which the classical Greeks described as gnomonic (Fig. 1).
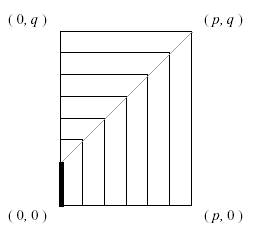
Fig. 1. Gnomonic diagram for the row (q - p). The illustration shows p = 6, q = 8. Note the dotted diagonal at 45° and the bold line of length 2 which defines row (2).
Now consider row (2):
Every second term, read as a fraction, may be reduced to its coprime form:
| 1/3, | 3/5, | 5/7, | 7/9, | ... | ||||
| 1/2, | 2/3, | 3/4, | 4/5, | ... |
These second terms belong to row (1), while the residual terms belong to a new kind of row in which the successor rule is
Treating p/q as a reducible fraction leads to complications. The notation lends itself to misinterpretations. Take as an example the term 9/19 given by Robinson and cited by Schindler. Its predecessor is 8/18, and its successor is 10/20. Suppose the terms represent the dimensions of a window, as Schindler imagines. It would be conventional to speak about the proportions of the window in terms of the ratios 4 : 9, 9 : 19, and 1 : 2. If the fractional terms, 8/18 and 10/20, are reduced to 4/9 and 1/2 respectively, then it will be seen that the predecessor comes from row (5) and the successor from row (1), whereas the original term 9/19 belongs to row (10).
To avoid such multiple interpretations in the remaining sections of this paper, the notation p x q will be used, where x is read as "by". This notation conforms to the architectural convention of dimensioning rooms, and corresponds to the rectangles in the gnomonic diagram, Fig. 1.
THE ELEMENTARY THEORY OF ROWS
The row arises in a
limited architectural context. A term p x
q is to be understood as the dimensions of a rectangular
space be it a room, a window opening, or a door. To remind us
of this spatial usage we call the term p x
q, a raum, with p designated as the width
and q as the length.
If ![]() is
a raum in a row, then the first raum of the row is 1 x
(q-p+1). The kth raum is then
is
a raum in a row, then the first raum of the row is 1 x
(q-p+1). The kth raum is then
k
x (q - p + k).
Call this the row (q - p). Any nth row may
be represented abstractly as the infinite set:
A designer may consider a space, or raum p x q. Within a space reference frame, the designer may enlarge, or reduce, the space by one module in one of two direction:
|
(p + 1) x (q + 1) |
(p + 1) x q |
(p + 1) x (q - 1) |
|
p x (q + 1) |
p x q |
p x (q - 1) |
|
(p - 1) x (q + 1) |
(p - 1) x q |
(p - 1) x (q - 1) |
In doing so the designer will move to neighboring rows:
| row(q - p) | row(q - p - 1) | row(q - p - 2) |
| row(q - p + 1) | row(q - p) | row(q - p - 1) |
| row(q - p + 2) | row(q - p + 1) | row(q - p) |
To generalize this; consider that p is changed by ±
a and all integers in between including 0, and q
by ± b similarly. Providing widths and lengths
are preserved, this gives (2a +1)(2b + 1) related
raum including the original:
|
(p + a) |
|
(q + b) | ... |
(p + a) |
|
q | ... |
(p + a) |
|
(q - b) |
|
|
|
: | ||||||||
|
p |
|
(q + b) | ... |
p |
x | q |
|
p |
x | (q - b) |
|
|
: | : | ||||||||
|
(p - a) |
|
(q + b) | ... |
(p - a) |
x | q |
|
(p - a) |
x | (q - b) |
ranging from row(q - p - a - b) to row(q - p + a + b) and all rows in between.
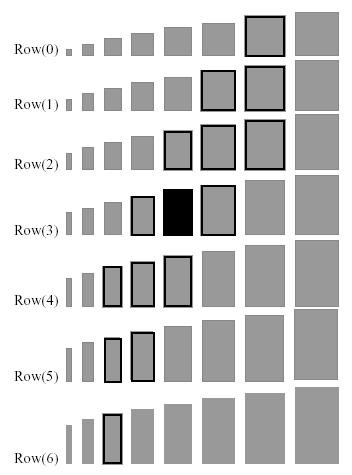
Except for row(1) the rows include rectangles in which the two dimensions are not coprime. That is, they share a common factor. Thus in row(2), the rectangle 2x4 has the same ratio as the rectangle 1x2 in row(1). It is useful to extract the coprime rectangles in which the two dimensions are prime to one another as shown in Table 1.
|
every term is coprime 1x2, 2x3, 3x4, 4x5, 5x6, 6x7, 7x8, 8x9, 9x10, 10x11, 11x12, ... |
|
eliminate every 2nd term to extract coprime terms. 1x3, 3x5, 5x7, 7x9, 9x11, 11x13, 13x15, 15x17, 17x19 , .. |
|
eliminate every 3rd term to extract coprime terms. 1x4, 2x5, 4x7, 5x8, 7x10, 8x11, 10x13, 11x14, 13x16, ... |
|
eliminate every 2nd term to extract coprime terms. 1x5, 3x7, 5x9, 7x11, 9x13, 11x15, 13x17, 15x19, 17x21, ... |
|
eliminate every 5th term to extract coprime terms. 1x6, 2x7, 3x8, 4x9, 6x11, 7x12, 8x13, 9x14, 11x16, 12x17, ... |
|
eliminate every 2nd and 3rd term to extract coprime terms. 1x7, 5x11, 7x13, 11x17, 13x20, 17x23, 19x25, 23x29, ... |
|
eliminate every 7th term to extract coprime terms. 1x8, 2x9, 3x10, 4x11, 5x12, 6x13, 8x15, ... 13x20, 15x22, .. |
|
eliminate every 3rd term to extract coprime terms. 1x10, 2x11, 4x13, 5x14, 7x16, 8x17, 10x19, 11x20, 13x22, ... |
|
eliminate every 11th term to extract coprime terms. 1x12, 2x13, ... 9x20, 10x21, 12x23, 13x24, ... 21x32, 23x34, ... |
|
eliminate every 3rd and 5th term to extract coprime terms. 1x16, 2x17, 4x19, 7x22, 8x23, 11x26, 13x28, 14x29, 16x31, ... |
Table 1. Examples of coprime raum where the two dimensions are prime to one another.
Consider the simple row(n) commencing 1xn. The difference is n - 1. Suppose n - 1 has a prime factor u. Let n - 1 = uv. The kth raum in the row is (k, n + k - 1) = (k, k + uv). If k is a multiple of u, say uw, then the kth term may be written (uw, uw + uv) = (uv, u (v+w)). Hence if the difference has a prime factor u, every uth term will be a non-prime term. This will be true for all prime factors of the difference. Eliminating all non-coprime terms will leave just the terms of the coprime row. All terms of coprime rows are based on a single unit module. The non-coprime terms from the simple rows are based on multiple units according the their positions in the simple row.
This may be seen in an example using a graphic representation of anthyphairesis [Fowler 1999; March 1999a, b]. Simply put, this means subtracting successively the largest squares from a given rectangle and residuals until the procedure stops. The procedure will not stop if the rectangle is incommensurable, but this is not the case here. For example, consider the partial diagram of row(6): 1x7, ... 11x17 (Fig. 3).
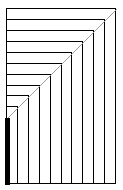
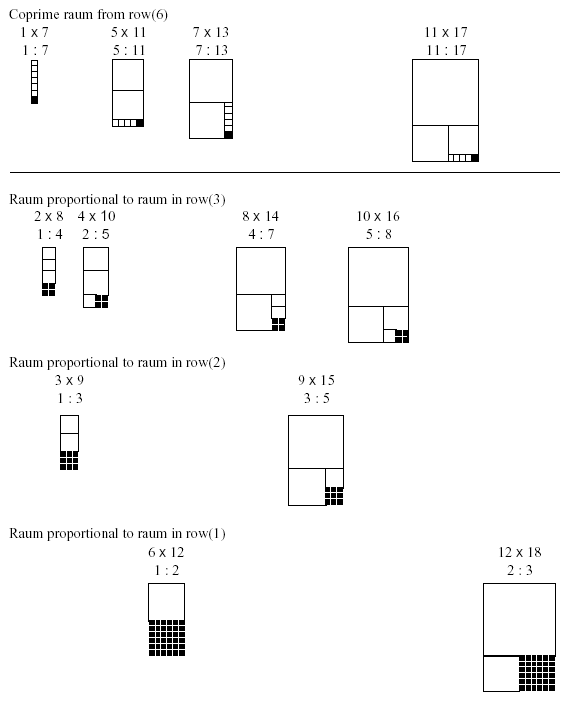
Successive subtraction of squares in these rectangles reveals the natural unit of each. Fig. 4 illustrates both the coprime and non-coprime rectangles of row(6).
It can be seen that a particular row may contain raum proportional to raum in other rows, as in this example. The question may be asked: when does a raum have the proportion of the root ratio r : s, that is a ratio in its coprime form? Consider the kth raum k x (n + k) from row(n). The required condition will be satisfied if r / s = k / (n + k), or if k = r n / (s - r). Now, k is an integer so that (s-r) must be a factor of r n for the condition to be met. This requires
If (s - r) = 1, that is r / s is subsuperparticular, or r x s is a raum in row(1), it will be seen that every row contains a raum proportional to r : s. That is, every row contains one raum proportional to 1 : 2, the double square, 2 : 3, 3 : 4, 4 : 5, ... .
For example, in row(6), the raum proportional to 1 : 2 is given by k = 1.6 / (2 - 1) = 6 and proportional to 2 : 3 is given by k = 2.6 / (3 - 2) = 12 (Fig. 4):
Yet there is no raum proportional to 5 : 9 in row(6) since
5.6 modulo (9 - 5) = 30 modulo ![]() .
.
According to Schindler, the unit of dimension, or module, is the choice of the architect. "He needs a unit dimension which is large enough to give his building scale, rhythm and cohesion. And last, but most important for the 'space architect,' it must be a unit which he can carry palpably in his mind in order to be able to deal with space forms freely but accurately in his imagination" [Schindler 1946; see Park 2003].
Robinson [1899: 298] gives an example of dimensional change using the method of regulating lines. He states that the "fundamental idea of proportion ... is that all parts share the same general character - what geometricians call "similar"; that is, that if one part is seven wide and ten high another part that is only eight high shall be about, or exactly, five and six tenths wide" (Fig. 5).
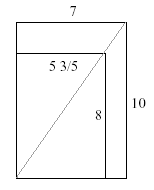
This represents a linear scale change of 80%; but Robinson does not take the opportunity to point this out.
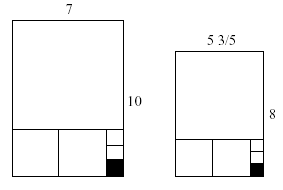
Fig. 6. The geometric method gives rise to scale changes such as this 80% linear reduction in the unit module.
In Fig. 6, the method of anthyphairesis, successive subtraction, reveals the "natural" unit of measure, or module of a rectangle [March 1999a, b]. This so called "geometric" method leads to two problems for Schindler. First, there is the question of uncertain scale and choice of unit. Second, there is the potential for unacceptable computation "for easy grasp." Schindler sees no good reason to accept a fraction such as 3/5. If, in figure 6, the unit is presumed to be 1 ft., then the reduced rectangle would have the dimensions 8 ft. by 5 ft. 7 1/5 in. which "it is hardly possible to visualize."
By using the 48 inch unit, Schindler proposed that it is possible to "feel" the larger rectangle as 1 3/4 x 2 1/2 units, and the smaller as either 1 1/2 x 2 units, or 1 1/3 x 2 units. This degree of refinement of the dimensions allows the architect "to carry in his mind" the design concept.
MODULAR COORDINATION
Schindler corresponded with the Chairman,
Frederick Heath Jr., of the U.S. Producers' Council, Subcommittee
on Modular Products, from 1944. His then unpublished "Reference
Frames in Space" [1946] was duplicated and circulated to
the Subcommittee on Building Layouts. The meeting which considered
Schindler's paper agreed on the term "modular coordination"
for product standardization. In a letter to Schindler, Heath
wrote:
It is recognized that through your years of experience you have made a substantial contribution to the art of modular planning. You refer to the 4 in. module as 'texture' and the 4 ft. as 'rhythm'. That expression was helpful to the Committee, and there was general agreement that we are dealing with texture rather than rhythm.
Schindler's paper was positively reviewed in Moduletter 38, the official notice of the Subcommittee.
An illustration will demonstrate the problem of employing the principle of geometric similitude with a modular grid. Suppose a ratio of 3 : 5 is adopted. The first modular rectangle will be 3M x 5M, where M is the chosen module. If the length is extended to 4M, the width will fall off the grid. The same is true for lengths of 5M, 6M, 7M, 8M, and 9MM. Only when the length 10M is reached does the width 6M fall on the grid. It is easy to see that only rectangles 3kM x 5kM, for integer values of k, fall wholly on the grid (Fig. 7).
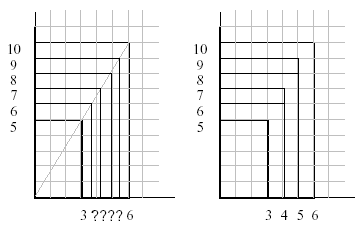
This example employs a ratio, 3 : 5, that Schindler recognized as a rational approximation to the golden section, although he rejected the value of the section in architectural design. Nevertheless, the example serves to introduce Le Modulor in which this ratio appears in the Fibonacci sequence of proportions promoted by Le Corbusier (Figure 8):
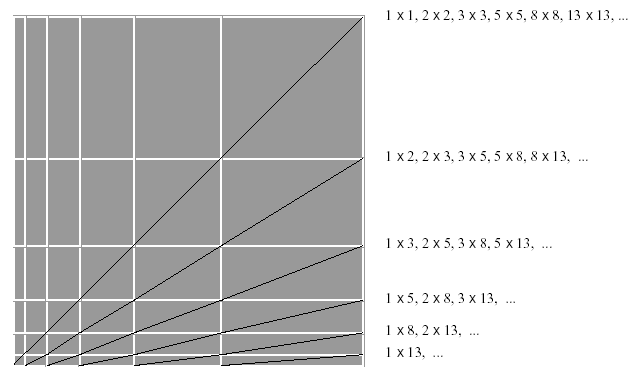
It comes as no surprise that Le Corbusier's proportional palette is a subset of Schindler's universal set {row(k) | k = 0, 1, 2, ... }. It may be of marginal interest that the first sequence -- from top to bottom -- in Figure 8 is row(0); that the next diagonal of rectangles come from row(1), row(1), row(2), row(3), row(5), ...; the next from row(2), row(3), row(5), row(8), ...; the next from row(4), row(6), row(10), ...; then row(7), row(11); and finally row(12). If the diagram is extended it will be found that the raum along the diagonals all come from Fibonacci sequences of rows:
| Rows | (1), (1), (2), (3), (5), (8), (13), ... |
| (2), (3), (5), (8), (13), (21), (34), ... | |
| (4), (6), (10), (16), (26), (42), .... | |
| (7), (11), (18), (29), (47), (76), ... | |
| (12), (19), (31), (50), (81), ... |
Schindler sought freedom from these artificial constraints and limitations. Proportion as such was not the issue. He was more concerned with the preservation of scale throughout a work, the rhythmic relationships and the play of the unit system. Where necessary the system could be broken. Consistency was no virtue. The grid did not have to be square. "It is not necessary that the designer be completely enslaved by the grid. I have found that occasionally a space-form may be improved by deviating slightly from the unit. Such sparing deviation does not invalidate the system as a whole but merely reveals the limits inherent in all mechanical schemes" [Schindler 1946].
IMPERIAL AND METRIC
Schindler,
like his exact contemporary Le Corbusier, was no enthusiast of
the metric system for architectural purposes. In the correspondence
with the Producers' Council, Heath sent Schindler a paper proposing
a 40 in. unit module arguing that within building tolerances
it was equivalent to a meter. For a quarter of a century, Schindler
had used 48 in. with few exceptions. The 40 in. module may be
divided by six factors 2, 4, 5, 8, 10, 20: the 48 in. module
by eight factor 2, 3, 4, 6, 8, 12, 16, 24. The metric equivalent
of 40 in. is 100 cm. which can be divided by the seven factors
2, 4, 5, 10, 20, 25, 50. For practical purposes these metric
measures include 2 in., 4 in., 8 in., 10 in., and 20 in. Imperial
equivalents. The metric equivalent to 48 in. is 120 cm. Now 120
is the ninth Ramanujan "highly composite" number defined
as a number that counting from 1 sets a record for the number
of its divisors. 120 is the first number with 16 divisors including
1 and 120. The factors can be represented on a modular lattice,
Fig. 9.
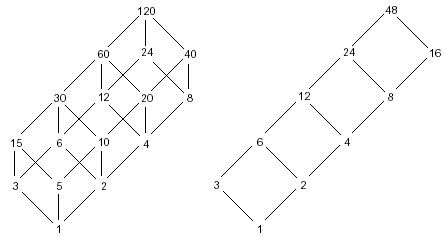
Figure 9. Modular lattices showing, left, the factors of 120 and, right, the factors of 48.
BACKGROUND
My interest in modular coordination
and proportional systems goes back to my collaboration
with Philip Steadman, who was largely responsible for the chapters
"Modules and numbers" and
"Proportion and series" in The Geometry of Environment
[March and Steadman 1971: 199-241].
In [March 1981], I wrote a short note on a certain class of tartan
grids which I had made use of
in previous design work.
I have written about Schindler's dimensioning system in several papers [March 1993a, b, c; 1999a]. I was unaware of Schindler's lecture notes at the time of writing. Judith Sheine first pointed out to me the sequence 1/2, 2/3. 3/4.4/5, ... and the musical scale which she had come across in Schindler's notes when researching her monograph [Sheine, 2001]. She believed it confirmed my emphasis on the musical analogy in Schindler's work. In the process of his doctoral investigation, Jin-Ho Park came across the same notebook and made the valuable connection with Robinson's articles [1898-99]. This source material has revised my views. I am no longer so convinced by the musical analogy in the form that I first promoted it. Any ratio involving small numbers will reproduce the same ratios to be found in musical theory. I was aware of this [1993a: 94-5]. Schindler's interest in Robinson's paper on proportion shown in his lecture notes suggests a more liberal appreciation of architectural proportions than adherence to musical harmony would permit. His approach is best explained arithmetically rather than geometrically, and his lecture notes confirm this.
I previously suggested that Schindler's system of dimensioning was "classical," and I believe this to be true. His son, Mark Schindler, remembers that his father would often relax in the evenings by examining "classical" architectural drawings. Schindler's lecture notes show a broad interest in architectural history. Where he introduces the notion of a modular unit and a "net for planning" based on the "largest common division," he sidebars ten dimensions of the thirteenth century Elisabethkirche, Marburg, as multiples of a 17 ft. unit. On the very next page he describes the concept of a "row." Again, it was Jin-Ho Park who brought the Schindler-Heath correspondence on modular coordination to my attention.
In Architectonics of Humanism [March 1998], I explained the classical understanding and application of ratios and proportion. The Greek gnomonic diagrams were illustrated there. Indeed, before I knew about Schindler's rows, I had speculated that certain temple platforms may have been derived by gnomonic methods. The platform of the Parthenon may be thought of as being generated from a 1x10 rectangle by gnomonic additions. That this base rectangle contains both the divine monad, 1, and the Pythagorean decad, 10, is surely no coincidence. These observation support the points made by Hendrik Berlage cited in Park [2003], Figure 10. In subsequent papers [March 1999b, c] I took up David Fowler's reconstruction of the mathematics associated with Plato's academy [1999], and related it to the pictorial methods characteristic of modern "shape grammars." This is where I employed the anthyphairetic representation of ratios.
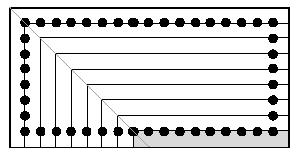
Figure 10. The platform of the Parthenon seen as a gnomonic diagram generated from a 1x 10 rectangle, tinted. The gnomonic rectangles belong to Schindler's row(9).
REFERENCES
Fowler, David. 1999. The Mathematics of Plato's
Academy. 2nd ed. London: Oxford University Press.
March, Lionel. 1981. A class of grids. Environment and Planning B 8: 325-332.
______. 1994a. Dr. How's magical music box. Chapter 12, pp. 124-145 in R.M. Schindler: Composition and Construction, Lionel March and Judith Scheine, eds. London: Academy Editions; Berlin: Ernst & Sohn.
______. 1994b. Log house, urhütte, and temple. Chapter 10, pp. 102-113 in R.M. Schindler: Composition and Construction, Lionel March and Judith Scheine, eds. London: Academy Editions; Berlin: Ernst & Sohn.
______. 1994c. 'Proportion is an alive and expressive tool...'. Chapter 9, pp. 88-101 in R.M. Schindler: Composition and Construction, Lionel March and Judith Scheine, eds. London: Academy Editions; Berlin: Ernst & Sohn.
______. 1998. Architectonics of Humanism: Essays on Number in Architecture. London: Academy Editions / John Wiley & Sons.
______. 1999a. Architectonics of proportion: a shape grammatical depiction of classical theory. Planning and Design 26: 91-100.
______. 1999b. Architectonics of proportion: historical and mathematical grounds. Planning and Design 26: 447-454.
______. 1999c. Music for the eyes: Schindler in proportion. Kenchiku Bunka 54 (635): 138-143. (In Japanese.)
March, Lionel and Judith Sheine, eds. 1998. R.M. Schindler: Composition and Construction. London: Academy Editions, Berlin: Ernst & Sohn.
March, Lionel and Philip Steadman. 1971. The Geometry of Environment. London: RIBA Publications; University Paperback, London: Methuen, 1975; Cambridge MA: The M.I.T. Press, 1975.
______. 1975. Geometria az Epiteszetben. Budapest: Muszaki Konyvkiadio. (Hungarian edition of The Geometry of Environment.)
______. 1975. Geometria dell'ambiente. Milan: Mazotta. (Italian edition of The Geometry of Environment.)
Park, Jin-Ho. 2003. Rudolph M. Schindler: Proportion, Scale and the "Row". Nexus Network Journal 5 (Autumn 2003).
Robinson, John Beverley. 1898-99. Principles for Architectural Composition. The Architectural Record VIII, 1: 1-25; 2:181-223; 3: 297-331; 4: 434-465.
Sarnitz, August. 1988. R. M. Schindler, Architect 1887-1953. New York: Rizzoli.
Schindler, Rudolph M. 1946. Reference Frames in Space. Architect and Engineer 165: 10, 40, 44-45. Also: pp. 57-61 in Lionel March and Judith Scheine, eds., R.M. Schindler: Composition and Construction, London: Academy Editions, Berlin: Ernst & Sohn; pp. 59-60 in August Sarnitz, R. M. Schindler, Architect 1887-1953, New York: Rizzoli.
Sheine, Judith. 2001. R. M. Schindler. Oxford: Phaidon Press.
ABOUT THE AUTHOR
On the personal recommendation
of Alan Turing, Lionel
March was admitted to Magdalene College, Cambridge, to
read mathematics under Dennis Babbage. There he gained a first
class degree in mathematics and architecture while taking an
active part in Cambridge theater life. In the early sixties,
he was awarded an Harkness Fellowship of the Commonwealth Fund
at the Joint Center for Urban Studies, Harvard University and
Massachusetts Institute of Technology under the directorships
of Martin Meyerson and James Q Wilson. He returned to Cambridge
and joined Sir Leslie Martin and Sir Colin Buchanan in preparing
a plan for a national and government center for Whitehall. He
was the first Director of the Centre for Land Use and Built Form
Studies, now the Martin Centre for Architectural and Urban Studies,
Cambridge University. As founding Chairman of the Board of the
private computer-aided design company, he and his colleagues
were among the first contributors to the 'Cambridge Phenomenon'
- the dissemination of Cambridge scholarship into high-tech industries.
In 1978, he was awarded the Doctor of Science degree for mathematical
and computational studies related to contemporary architectural
and urban problems.
Before coming to Los Angeles he was Rector and Vice-Provost of
the Royal College of Art, London. During his Rectorship he served
as a Governor of Imperial College of Science and Technology.
He has held full Professorships in Systems Engineering at the
University of Waterloo, Ontario; and in Design Technology at
The Open University, Milton Keynes. At The Open University, as
Chair, he doubled the faculty in Design and established the Centre
for Configurational Studies. He came to UCLA in 1984 as a Professor
in the Graduate School of Architecture and Urban Planning. He
was Chair of Architecture and Urban Design from 1985-91. He is
currently Professor in Design and Computation and a member of
the Center for Medieval and Renaissance Studies. He was a member
of UCLA's Council on Academic Personnel from 1993, and its Chair
for 1995/6. He is a General Editor of Cambridge Architectural
and Urban Studies, and Founding Editor of the journal Planning
and Design. The journal is one of four sections of Environment
and Planning, which stands at "the top of the citation
indexes." Among the books he has authored and edited are:
The Geometry of Environment, Urban Space and Structures,
The Architecture of Form, and R. M. Schindler: Composition
and Construction. His most recent research publications include:
"The smallest interesting world?", "Babbage's
miraculous computation revisited," "Rulebound unruliness,"
"Renaissance mathematics and architectural proportion in
Alberti's De re aedificatoria," and "Architectonics
of proportion: a shape grammatical depiction of classical theory."
His book Architectonics of Humanism: Essays on Number in Architecture
before The First Moderns, a companion volume to Rudolf Wittkower's
Architectural Principles in the Age of Humanism was published,
together with a new edition of the Wittkower, in the Fall 1998.
|
Lionel March, "Rudolph M. Schinder: Space Reference Frame, Modular Coordination, and the 'Row'", Nexus Network Journal, vol. 5 no. 2 (Autumn 2003), http://www.nexusjournal.com/March-v5n2.html |

Copyright ©2003 Kim Williams Books
