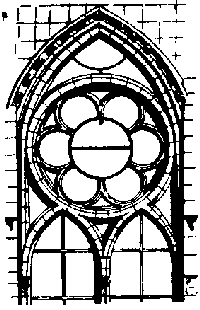
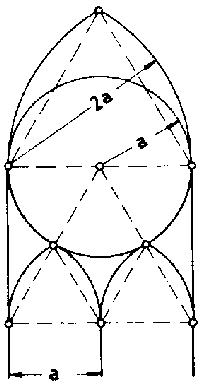
One of the most typical elements of Gothic architecture is the tracery found in windows, on walls, and in many other places in Gothic churches. What is mathematical about it? Tracery is exclusively constructed from circular arcs and straight line segments! It is the most mathematical kind of art known to me. In many of the thousands of Gothic churches and other buildings of that time surviving in Europe you can find nice examples, take photos and analyze them geometrically at home.
Traceries appear some 60 years after the first examples of Gothic
churches in the 1200's in Reims. There their construction is,
like that of the typical pointed Gothic window, based on the
equilateral triangle. In the course of stylistic development,
the constructions became more and more elaborate and less determined
by geometry until we find whole windows covered by wavy ornaments
in the flamboyant late Gothic of about 1500.
Much more than the usual geometric designs of traceries can be
found in the cloisters of the Cistercian monastery of Hauterive
near Fribourg, Switzerland. Here the theme of the windows is
geometry itself. Regular n-gons are shown for n = 3,4,5,6 and
8. Variations of the pentagon show the pentagram and a delicately
constructed rose. The whole cloisters seems to be a commentary
to Euclid's book IV on the regular n-gons carved in stone.