We study the uniform distribution of the polynomial sequence
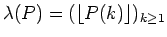
modulo integers, where
P(
x) is a polynomial with real coefficients. In the nonlinear case, we
show that
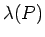
is uniformly distributed in
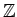
if and
only if
P(
x) has at least one irrational coefficient other than the
constant term. In the case of even degree, we prove a stronger result:
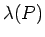
intersects every congruence class modulo every integer if
and only if
P(
x) has at least one irrational coefficient other than
the constant term.
Received September 11 2018; revised version received December 16 2018.
Published in Journal of Integer Sequences,
December 17 2018.

