 |
Journal of Integer Sequences, Vol. 20 (2017), Article 17.8.2 |
 |
Journal of Integer Sequences, Vol. 20 (2017), Article 17.8.2 |
Abstract:
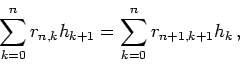
(Concerned with sequences
A000045
A000073
A000078
A000085
A000108
A000110
A000255
A000262
A000932
A000984
A001591
A001592
A001764
A002720
A002793
A004319
A006629
A006630
A006631
A007318
A007405
A008277
A008287
A025174
A027907
A035343
A040027
A049425
A063260
A063265
A066178
A079262
A102594
A102893
A105287
A122189
A131689
A132393
A171890
A213651
A213652
A230547
A233657
A236194.)
Received September 28 2016; revised versions received October 3 2016; July 6 2017; August 24 2017; August 25 2017. Published in Journal of Integer Sequences, August 31 2017.