Integer Sequences, Functions of Slow Increase, and the Bell Numbers

In this article we first prove a general theorem on integer sequences

such that the following asymptotic formula holds,

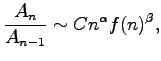
$\displaystyle \frac{A_{n}}{A_{n-1}}\sim C n^{\alpha} f(n)^{\beta},$

where

is a function of slow increase,

, $\alpha>0$ and $\beta$ is a real number.

We also obtain some results on the Bell numbers using well-known formulae. We compare the Bell numbers with and $(0<h\leq 1)$ .

Finally, applying the general statements proved in the article we obtain the formula

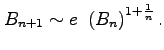
$\displaystyle B_{n+1}\sim e\ \left(B_n\right)^{1+\frac{1}{n}}.$