André proved that
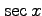
is the generating
function of all up-down permutations of even length and
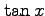
is the generating function of all up-down permutation
of odd length. There are three equivalent ways to define
up-down permutations in the symmetric group
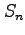
. That is, a permutation
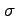
in the symmetric group
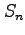
is an
up-down permutation if either (i) the rise set of
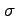
consists
of all the odd numbers less than
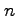
, (ii) the descent set of
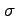
consists of all even number less than
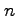
, or (iii) both (i) and (ii).
We consider analogues of André's results
for colored permutations of the form
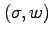
where
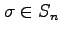
and
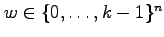
under the product order.
That is, we define
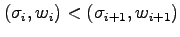
if and only if
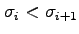
and
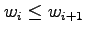
.
We then say a colored permutation
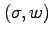
is
(I) an
up-not up permutation
if the rise set of
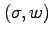
consists
of all the odd numbers less than
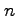
, (II) a
not down-down permutation
if the descent set of
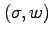
consists
of all the even numbers less than
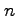
, (III) an
up-down permutation
if both (I) and (II) hold. For
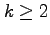
,
conditions (I), (II), and (III) are pairwise distinct. We
find
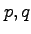
-analogues of the generating functions
for up-not up, not down-down, and up-down colored permutations.

