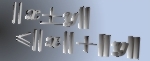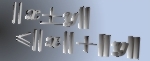| |
|
|
| |
Volume 2, Issue 1, Article 2 |
|
|
|
|
|
| |
Monotone Methods Applied to Some Higher Order Boundary Value Problems
|
| |
|
Authors: |
John M. Davis, Johnny Henderson, |
|
| |
|
Keywords:
|
Differential Inequality, Monotone Methods, Upper and Lower Solutions, Maximum Principle |
|
| |
|
Date Received:
|
26/06/00 |
|
| |
|
Date Accepted:
|
06/07/00 |
|
| |
|
Subject Codes: |
34B15,34A40,34C11,34C12
|
|
| |
|
Editors: |
Ravi P. Agarwal, |
|
| |
|
|
|
|
|
| |
|
Abstract: |
We prove the existence of a solution for the nonlinear boundary value problem
where ![$ f:[0,1]times{mathbb{R}}^{m+2}o{mathbb{R}}$](images/021_00_JIPAM/img3.gif) is continuous. The technique used here is a monotone method in the presence of upper and lower solutions. We introduce a new maximum principle which generalizes one due to Bai which in turn was an improvement of a maximum principle by Ma.
|
|
|
|
|
|
| |
|
|
|
|
| |
|
|
|
|
|
Home
![$displaystyle u^{(2m+4)}=fleft(x,u,u'',dots,u^{(2m+2)}ight), qquad x in [0,1],$](images/021_00_JIPAM/img1.gif){kind=small}
